Two of the most important choices you will have to make when you take out a mortgage is the size of your deposit, and the term over which you would like to pay off the mortgage.
Why this matters
It matters, because your choices will impact you in three ways
- your monthly mortgage payments
- the amount of equity you build up over the years
- the loan to value percentage
We will show you with an example how your choices may affect you in the present and in the future.
Our example
We will use an “average” first time buyer to illustrate.. Lucky for us there is lots of data that tells us what average looks like – the average price for a property in the UK is around £300,000, and the average salary for someone in full time employment is around £35,000.
Assume our buyer is an “average couple” with a joint income of around £70,000. This means they should be able to borrow somewhere between £280,000 and £310,000. So with their deposit, they should be able to buy a property for £300,000.
Lets then assume they only have two choices to make:
Deposit -should they put down £15,000 (5%) or £30,000 (10%) as a deposit?
Mortgage Term – should they repay the mortgage over 25 years or 30 years?
This is how their choice will affect their monthly payments, their equity and their loan to value:
Please note all calculations are illustrative and based on assumptions – in this case the two most important assumptions are the mortgage interest rate and the projected property growth. To keep the article simple, the data used in all the calculations are included at the end of the article.
A higher deposit and a longer term reduce your monthly payments (a lot)
A 30 year / 10% mortgage comes to over £250 per month less than a 25 year / 5% mortgage. No need to explain the significance of this. Also interesting that in this scenario, a shorter term and a bigger deposit stacks up quite evenly with a smaller deposit and a longer term.
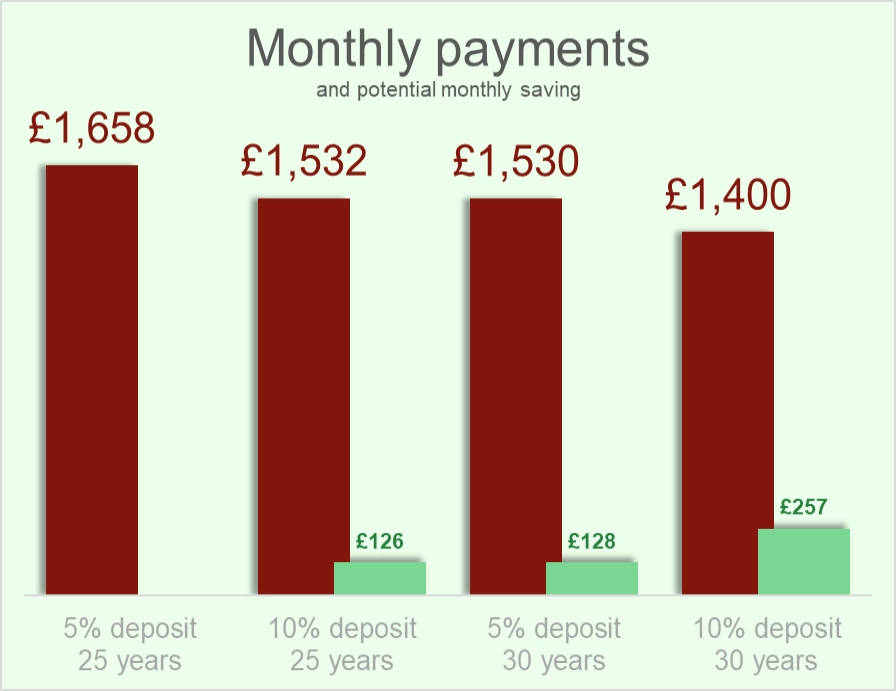
If you want to reduce your monthly payments as much as possible, go for the longer mortgage with a bigger deposit…
A bigger initial deposit helps to secure a better deal when you remortgage
Your mortgage would usually have a fixed interest rate for the first 2, 3 or 5 years, and at the end of this period you would remortgage with your current lender or with a new one (it always pays to shop around). It is important to get yourself in a position to negotiate a good deal when you renew.
If your new loan amount is less than 80% of the value of the property, you should be able to get a much better interest rate and consequently much lower monthly repayments (the difference could amount to a few hundred pounds per month).
Lets see how the choice of mortgage term and initial deposit affects loan to value after three years.
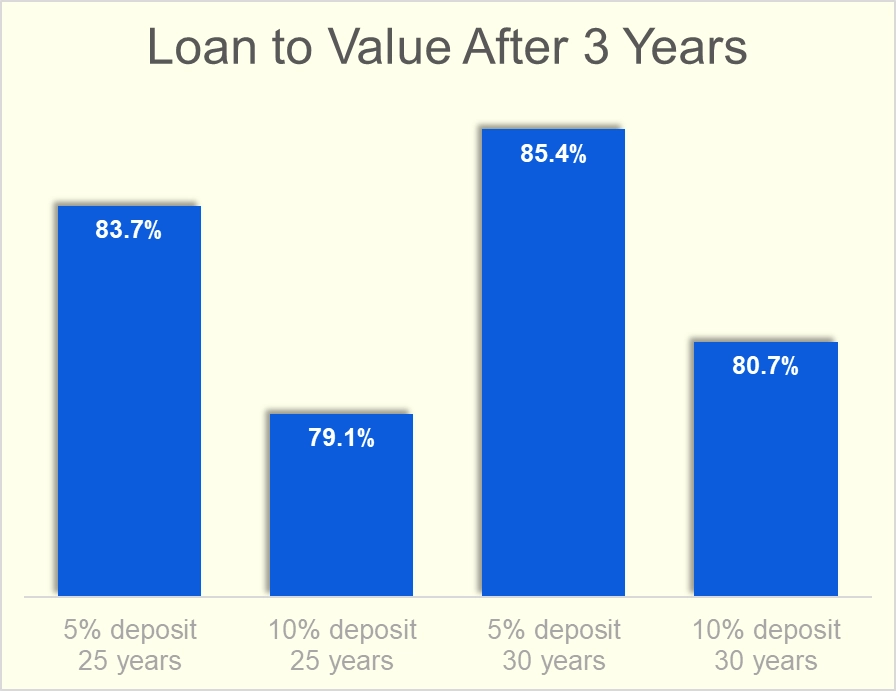
The two 10% deposit options are just about on the 80% LTV mark, and the 5% deposit options don’t quite get there – bottom line, a higher deposit will reduce your monthly payments, AND increase your chances on getting a good deal when you remortgage
The calculation above is based on an assumption that your property value will grow by 2% per year. In reality, the growth could be higher or lower. Over the past 5 years, average growth was around 5% per year, but beware of averages. It is best to look at historic data for properties similar to yours and in the same postcode area to get a more realistic view. Also important confirm with your broker or lender that they will revalue the property when you remortgage
5 years down the line, your choice matter (slightly) less
The final perspective to consider is the amount of equity you will have after some years. Equity is the amount of cash you will have left should you sell your property and settle the mortgage with the proceeds (when you hear the term negative equity, it means the house is worth less than the outstanding balance on the mortgage)
Your equity could become a very important factor should you decide to upsize or move home after 5 years. So lets see how much equity our hypothetical buyer might have after 5 years
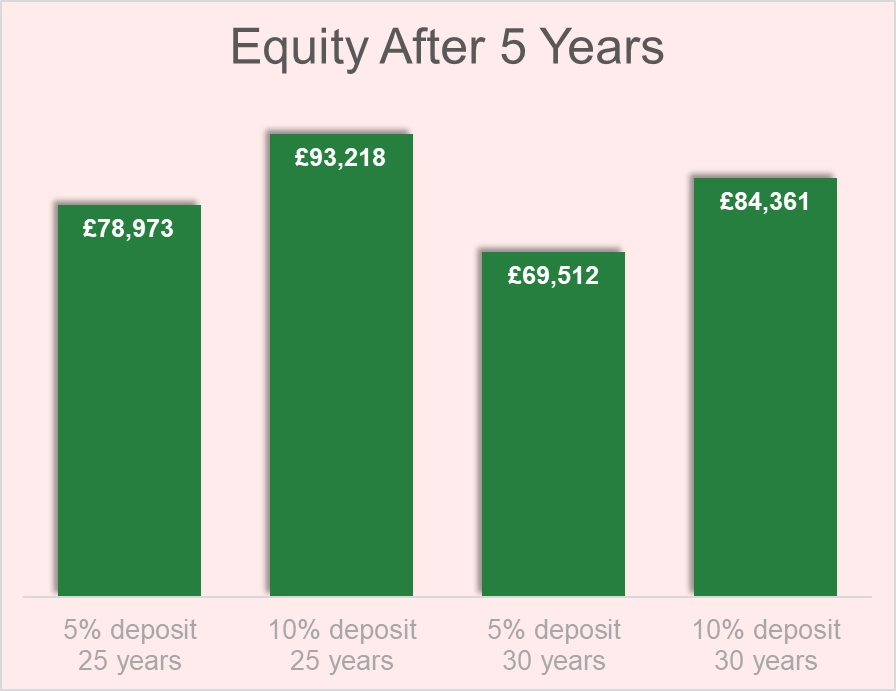
With the equity in this example and the amount a lender might be willing to lend to our hypothetical couple, in 5 year’s time they should be able to afford a house somewhere between £470,000 and £490,000 (just remember that stamp duty will be payable on a second purchase – around £14,000 to £15,000 in this example). So really not a huge difference, but still worth knowing
Use our calculator with your own numbers
Mortgage Repayment Calculator
The geeky bits – underlying data
All calculations are based on assumptions. For the sake of simplicity Here our underlying data – reach out to us on Facebook if you have more questions or comments
| 5% deposit over 25 years | 10% deposit over 25 years | 5% deposit over 30 years | 10% deposit over 30 years | |
| Household Income | £70,000.00 | £70,000.00 | £70,000.00 | £70,000.00 |
| Max loan amount | £315,000.00 | £315,000.00 | £315,000.00 | £315,000.00 |
| Monthly payment | -£1,832.29 | -£1,786.82 | -£1,690.99 | -£1,633.71 |
| Purchase price | £300,000 | £300,000 | £300,000 | £300,000 |
| Deposit | 5% | 10% | 5% | 10% |
| Deposit Amount | £15,000 | £30,000 | £15,000 | £30,000 |
| 2 year fixed interest rate (will use this for all mortgage calculations) | 4.95% | 4.70% | 5.00% | 4.70% |
| 3 year fixed interest rate | 5.10% | 4.80% | 5.20% | 4.90% |
| 5 year fixed interest rate | 4.95% | 4.55% | 4.95% | 4.55% |
| Term | 25 | 25 | 30 | 30 |
| Loan Amount | £285,000 | £270,000 | £285,000 | £270,000 |
| Repayment | £1,658 | £1,532 | £1,530 | £1,400 |
| Monthly saving | £126 | £128 | £257 | |
| Balance after 2 years | £272,862.13 | £258,095.02 | £276,375.29 | £261,390.81 |
| Balance after 3 years | £266,328.42 | £251,710.37 | £271,729.24 | £256,773.70 |
| Balance after 5 years | £252,251.66 | £238,006.45 | £261,711.89 | £246,863.60 |
| Annual capital growth | 2% | 2% | 2% | 2% |
| Property value after 2 years | £312,120 | £312,120 | £312,120 | £312,120 |
| Property value after 3 years | £318,362 | £318,362 | £318,362 | £318,362 |
| Property value after 5 years | £331,224 | £331,224 | £331,224 | £331,224 |
| Equity after 2 years | £39,258 | £54,025 | £35,745 | £50,729 |
| Equity after 3 years | £52,034 | £66,652 | £46,633 | £61,589 |
| Equity after 5 years | £78,973 | £93,218 | £69,512 | £84,361 |
| Loan to value after 2 years | 87.42% | 82.69% | 88.55% | 83.75% |
| Loan to value after 3 years | 83.66% | 79.06% | 85.35% | 80.65% |
| Loan to value after 5 years | 76.16% | 71.86% | 79.01% | 74.53% |
| Using the released equity at the same deposit % after 2 years theoretically I can buy a property worth | £785,157 | £540,250 | £714,894 | £507,292 |
| Using the released equity at the same deposit % after 3 years theoretically I can buy a property worth | £1,040,680 | £666,520 | £932,663 | £615,887 |
| Using the released equity at the same deposit % after 5 years theoretically I can buy a property worth | £1,579,452 | £932,178 | £1,390,247 | £843,606 |
| Annual salary growth | 5% | 5% | 5% | 5% |
| With a salary of £77,175 you will get a loan for | £347,287.50 | £347,287.50 | £347,287.50 | £347,287.50 |
| With a salary of £81,034 you will get a loan for | £364,651.88 | £364,651.88 | £364,651.88 | £364,651.88 |
| With a salary of £89,340 you will get a loan for | £402,028.69 | £402,028.69 | £402,028.69 | £402,028.69 |
| Realistic estimated purchase power | ||||
| After 2 years – equity (less 2% costs) + max loan amount | £385,760.21 | £400,231.98 | £382,317.31 | £397,002.10 |
| After 3 years – equity (less 2% costs) + max loan amount | £415,645.18 | £429,970.87 | £410,352.37 | £425,008.80 |
| After 5 years – equity (less 2% costs) + max loan amount | £479,421.82 | £493,382.13 | £470,150.80 | £484,702.12 |
